¹H-enhanced ¹⁰³Rh NMR spectroscopy and relaxometry of ¹⁰³Rh(acac)₃ in solution

Recently developed polarisation transfer techniques are applied to the ¹⁰³Rh nuclear magnetic resonance (NMR) of the ¹⁰³Rh(acac)₃ coordination complex in solution. Four-bond ¹H–¹⁰³Rh J couplings of around 0.39 Hz are exploited to enhance the ¹⁰³Rh NMR signal and to estimate the ¹⁰³Rh T₁ and T₂ relaxation times as a function of field and temperature. The ¹⁰³Rh longitudinal T₁ relaxation in ¹⁰³Rh(acac)₃ is shown to be dominated by the spin–rotation mechanism, with an additional field-dependent contribution from the ¹⁰³Rh chemical shift anisotropy.

1 Introduction

Although rhodium is one of the few chemical elements with a 100 % abundant spin-$\mathrm{1}/\mathrm{2}$ isotope, the routine nuclear magnetic resonance (NMR) of ¹⁰³Rh has been inhibited by its very small gyromagnetic ratio, which is negative and ∼ 31.59 times less than that of ¹H (Mann, 1991).

While indirectly detected ¹⁰³Rh NMR has had an appreciable history (Crocker et al., 1979; Dykstra et al., 1981; Brevard and Schimpf, 1982; Heaton et al., 1983; Brevard et al., 1981; Herberhold et al., 1999; Ernsting et al., 2004; Carlton, 2008), advances in instrumentation and methodology have allowed rapid observation of ¹⁰³Rh NMR parameters on standard commercial NMR spectrometers, leading to a recent renaissance of the field (Chan et al., 2020; Bajo et al., 2020; Wiedemair et al., 2021; Widemann et al., 2021; Rösler et al., 2021; Sheng Loong Tan et al., 2021; Caló et al., 2021; Samultsev et al., 2022; Harbor-Collins et al., 2023, 2024; Holmes et al., 2023; Lutz et al., 2023).

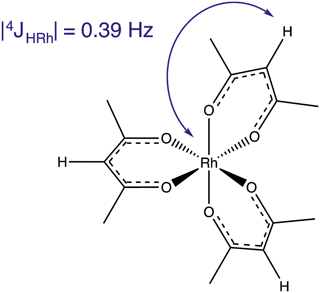
The rhodium  (III) acetylacetonate (¹⁰³Rh(acac)₃) complex (see Fig. 1) currently serves as the International Union of Pure and Applied Chemistry (IUPAC) ¹⁰³Rh NMR chemical-shift reference (Carlton, 2008). To the wider scientific community, ¹⁰³Rh(acac)₃ is better known for its role in the production of thin rhodium films and nanocrystals for use in catalysis (Zhang et al., 2007; Aaltonen et al., 2005; Choi et al., 2016).

The early studies of nuclear spin relaxation in ¹⁰³Rh(acac)₃ were greatly limited by the poor ¹⁰³Rh signal strength, and they provided somewhat conflicting conclusions for the ¹⁰³Rh relaxation mechanisms (Grüninger et al., 1980; Benn et al., 1985; Maurer et al., 1982). Recently, a two-bond ¹³C–¹⁰³Rh coupling of 1.1 Hz was observed in ¹⁰³Rh(acac)₃ and was exploited for triple-resonance experiments (Caló et al., 2021).

We now report the observation of a four-bond ¹H–¹⁰³Rh J coupling of |⁴J_HRh| ≃ 0.39 Hz between the central ¹⁰³Rh nucleus and each of the three methine ¹H nuclei in ¹⁰³Rh(acac)₃ (see Fig. 1). These small couplings are exploited for the ¹H-enhanced ¹⁰³Rh NMR spectroscopy of the ¹⁰³Rh(acac)₃ complex. ¹⁰³Rh spin–lattice T₁ and spin–spin T₂ relaxation time constants are measured over a range of magnetic fields and temperatures. The ¹⁰³Rh T₁ relaxation is found to be dominated by spin–rotation, with an additional contribution from the chemical shift anisotropy (CSA), which is significant at high fields.

Figure 1 The molecular structure of rhodium  (III) acetylacetonate, ¹⁰³Rh(acac)₃, which has point group symmetry D₃. This work exploits the long-range ⁴J_HRh scalar couplings for polarisation transfer between the ¹⁰³Rh and methine ¹H spins.

2 Experimental

Experiments were performed on a saturated (∼ 140 mM) solution of rhodium  (III) acetylacetonate (¹⁰³Rh(acac)₃) dissolved in 350 µL CDCl₃. ¹⁰³Rh(acac)₃ was purchased from Sigma-Aldrich and used as received. ¹⁰³Rh(acac)₃ is a bright yellow powder, which is dissolved in CDCl₃ to form a solution with a deep golden colour.

The radio frequency channels were additionally isolated by installing a bandpass (K&L Microwave) and low-pass (Chemagnetics 30 MHz) filter at the preamplifier outputs of the ¹H and ¹⁰³Rh channels, respectively. Pulse powers on the ¹H and ¹⁰³Rh channels were calibrated to give a matched nutation frequency of 2π × 4000 Hz, corresponding to a 90° pulse length of 62.5 µs. Field-cycling experiments were performed using a motorised fast-shuttling system (Zhukov et al., 2018; Harbor-Collins et al., 2023, 2024). The shuttling time was kept constant at 2 s, in both directions.

3 Results

3.1 ¹H spectrum

The ¹H spectrum for ¹⁰³Rh(acac)₃ in CDCl₃ (shown in Fig. 2a) features two resonances: a singlet at 2.170 ppm, corresponding to the six methyl protons on each acac ligand, and a broad, weak doublet centred at 5.511 ppm, corresponding to the acac methine protons. An expanded region showing just the methine resonance is presented in Fig. 2b. The four-bond ¹⁰³Rh–¹H spin–spin coupling is estimated to be |⁴J_HRh| = 0.39 ± 0.01 Hz.

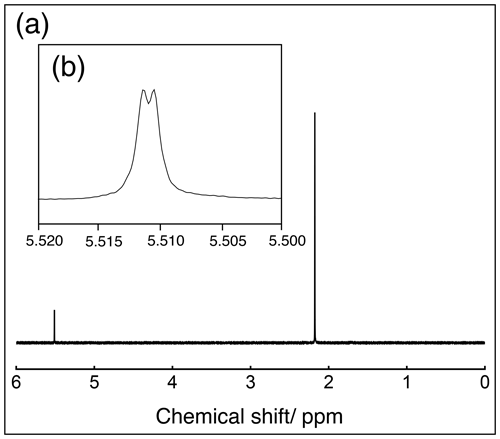
Figure 2 (a) ¹H spectrum of a ∼ 140 mM solution of ¹⁰³Rh(acac)₃ in CDCl₃, acquired at 9.4 T and 298 K, in a single transient. Lorentzian line broadening (1 Hz) was applied. (b) Expanded view of the methine ¹H resonance. Negative Lorentzian line broadening (−0.2 Hz) was applied to enhance the resolution.

3.2 ¹⁰³Rh spectra

3.2.1 Direct ¹⁰³Rh excitation

The ¹H-decoupled ¹⁰³Rh spectrum of the ¹⁰³Rh(acac)₃ solution, acquired with single-pulse excitation of ¹⁰³Rh transverse magnetisation, is shown in Fig. 3a and displays a single peak with the ¹⁰³Rh chemical shift of 8337.6 ppm. The signal-to-noise ratio is quite poor, even after 12 h of data acquisition.

Figure 3 ¹H-decoupled ¹⁰³Rh NMR spectra of a ∼ 140 mM solution of ¹⁰³Rh(acac)₃ in CDCl₃, in a field of 9.4 T and at a temperature of 295 K. Lorentzian line broadening (1 Hz) was applied to all spectra. ¹⁰³Rh chemical shifts are referenced to the absolute frequency (Ξ(¹⁰³Rh) = 3.16 %). In all spectra, ¹H decoupling was achieved using continuous wave decoupling with 0.05 W of power, corresponding to a nutation frequency of 1 kHz. (a) ¹⁰³RhHdec spectrum, acquired using 300 transients, each using a single ¹⁰³Rh 90° pulse. The waiting interval between transients was 150 s. The total experimental duration was ∼ 12 h. (b) ¹⁰³RhHdec spectrum, acquired using 16 transients and the pulse sequence shown in Fig. 4, with n=11 repetitions of the DualPol (dual-channel PulsePol) sequence. The waiting interval between transients was 18 s. The total experimental duration was 5 min. (c) ¹⁰³RhHdec spectrum, acquired using 16 transients and an optimised refocused-INEPT (Insensitive Nucleus Enhancement by Polarisation Transfer) sequence. The waiting interval between transients was 18 s. The total experimental duration was 5 min.

3.2.2 ¹H–¹⁰³Rh polarisation transfer by DualPol

Polarization transfer from the ¹H nuclei to the ¹⁰³Rh nuclei was performed using the previously described DualPol pulse sequence incorporating acoustic-ringing suppression (Harbor-Collins et al., 2023), as shown in Fig. 4.

Figure 4 Pulse sequence for the acquisition of ¹H-enhanced ¹⁰³Rh spectra; an expanded view of the DualPol pulse sequence module is shown at the bottom. The black rectangles indicate symmetrised BB1 composite 180° pulses (Wimperis, 1994; Cummins et al., 2003) and τ indicates an interpulse delay. Phase cycles are given by the following: ${\mathit{\varphi }}_{\mathrm{1}}=[-x,x,-x,x]$, ${\mathit{\varphi }}_{\mathrm{2}}=[x,x,-x,-x]$, ${\mathit{\varphi }}_{\mathrm{3}}=[x,x,x,x,y,y,y,y,-x,-x,-x,-x,-y,-y,-y,-y]$ and the receiver ${\mathit{\varphi }}_{\text{rec}}=[x,-x,x,-x,y,-y,y,-y,-x,x,-x,x,-y,y$,$-y,y]$.

The DualPol sequence consists of two synchronised PulsePol sequences (Schwartz et al., 2018), applied simultaneously on two radio frequency channels.

The PulsePol sequence was originally developed in the context of electron–nucleus polarisation transfer (Schwartz et al., 2018). As discussed in Sabba et al. (2022), PulsePol may be interpreted as a “riffled” implementation of an R sequence, using the nomenclature of symmetry-based recoupling in solid-state NMR (Carravetta et al., 2000). In the case of PulsePol, the R element is a composite 90_y180_x90_y pulse, with “windows” inserted between the pulses. Furthermore, in the current implementation, the central 180_x pulse of each R element is itself substituted by a BB1 composite pulse (Wimperis, 1994). That substitution was previously shown to increase the robustness of the pulse sequence with respect to deviations in the radio frequency amplitudes and resonance offsets (Sabba et al., 2022). The total R-element duration, including all pulses and windows, is denoted using τ_R here (see Fig. 4).

For the experiments described here, the DualPol sequences used an R-element duration equal to τ_R = 70 ms, with pulse durations given by τ₉₀ = 62.5 µs for the 90° pulses and τ_BB1 = 10 × τ₉₀ = 625 µs for the BB1 composite 180° pulses.

The ¹⁰³Rh and methine ¹H nuclei of ¹⁰³Rh(acac)₃ form an I₃ S spin system, where the ¹⁰³Rh nucleus is the S spin and the magnetically equivalent ¹H nuclei are the I spins.

The DualPol spin dynamics are identical to those for Hartmann–Hahn J cross-polarisation (Chingas et al., 1981). The DualPol average Hamiltonian has the following form:

$$\begin{array}{}\text{(1)}& {\stackrel{\mathrm{‾}}{H}}^{\left(\mathrm{1}\right)}=\mathit{\kappa }\times \mathrm{2}\mathit{\pi }{J}_{\text{IS}}({\mathrm{I}}_x{S}_x+{\mathrm{I}}_y{S}_y),\end{array}$$

where the scaling factor is given by $\mathit{\kappa }=\mathrm{1}/\mathrm{2}$ in the limit of short, ideal, radio frequency pulses. In the absence of relaxation and pulse imperfections, a DualPol sequence with a scaling factor $\mathit{\kappa }=\mathrm{1}/\mathrm{2}$, applied to an I₃ S spin system should give rise to the following enhancement of the S-spin magnetisation, relative to its thermal-equilibrium value:

$$\begin{array}{}\text{(2)}& \begin{array}{rl}{\mathit{ϵ}}_{\text{DualPol}}\left(T\right)=& {\displaystyle \frac{{\mathit{\gamma }}_{\mathrm{I}}}{\mathrm{4}{\mathit{\gamma }}_{\mathrm{S}}}}\left\{\mathrm{2}{\sin }^{\mathrm{2}}\left(\frac{\mathrm{1}}{\mathrm{2}}{J}_{\text{IS}}T\right)\right.\\ & \left.+{\sin }^{\mathrm{2}}\left(\mathit{\pi }{J}_{\text{IS}}T\right)+\mathrm{2}{\sin }^{\mathrm{2}}\left(\frac{\mathrm{1}}{\mathrm{2}}\sqrt{\mathrm{3}}\mathit{\pi }{J}_{\text{IS}}T\right)\right\}.\end{array}\end{array}$$

Here, T is the overall duration of the DualPol sequence. As the magnetogyric ratios of ¹⁰³Rh and ¹H have opposite signs, the function ϵ_DualPol(T) is negative for all values of T. The blue curve in Fig. 5 shows a plot of |ϵ_DualPol| against T, for a J coupling of |J_IS| ≃ 0.39 Hz. The maximum value of |ϵ_DualPol| is given in the absence of relaxation by

$$\begin{array}{}\text{(3)}& \left|{\mathit{ϵ}}_{\text{DualPol}}^{\text{max}}\right|=\mathrm{|}\mathrm{17}{\mathit{\gamma }}_{\mathrm{I}}/\mathrm{16}{\mathit{\gamma }}_{\mathrm{S}}\mathrm{|}\simeq \mathrm{33.56}\end{array}$$

for the case of I = ¹H and S = ¹⁰³Rh. As Eq. (2) is quasi-periodic (Chingas et al., 1981), the value of T which maximises |ϵ_DualPol| is indeterminate. The first maximum may be found numerically and occurs at the duration T_1st max ≃ $\mathrm{0.6098}{J}_{\text{IS}}^{-\mathrm{1}}$, at which point the theoretical enhancement is given by |ϵ_DualPol| ≃ $\mathrm{1.052}\mathrm{|}{\mathit{\gamma }}_{\mathrm{I}}/{\mathit{\gamma }}_{\mathrm{S}}\mathrm{|}$ ≃ 33.23 for the case of I = ¹H and S = ¹⁰³Rh. Hence, for the estimated ¹H–¹⁰³Rh J coupling of |J_IS|≃ 0.39 Hz, assuming $\mathit{\kappa }=\mathrm{1}/\mathrm{2}$, the ¹⁰³Rh signal enhancement is expected to reach its first maximum at a DualPol duration of T_1st max ≃ 1.563 s, in the absence of relaxation.

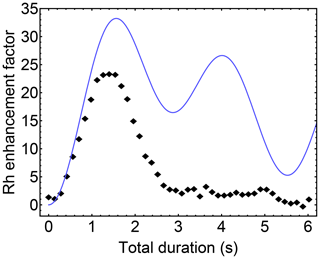
Figure 5 ¹⁰³Rh signal enhancement factor for ¹⁰³Rh(acac)₃ as a function of DualPol sequence duration T, normalised against thermal-equilibrium ¹⁰³Rh polarisation. Black diamonds: experimental data points; solid blue line: the theoretical enhancement factor |ϵ_DualPol(T)| for an I₃ S spin system in the absence of relaxation, as given by Eq. (2) for |J_IS| = 0.39 Hz.

In the experiments described here, the optimum duration of the DualPol sequence was found for a repetition number of n=11. For an R-element duration of τ_R = 70 ms, this corresponds to a total DualPol sequence duration of T = 1.54 s, which is in good agreement with the theoretical value.

The DualPol-enhanced ¹⁰³Rh spectrum is shown in Fig. 3b and displays an experimental signal enhancement of ∼ 23 over the directly excited ¹⁰³Rh spectrum in Fig. 3a.

Figure 5 shows the experimental ¹⁰³Rh signal enhancement factor as a function of the DualPol sequence duration T. Although the maximum of the experimental enhancement occurs at a similar position to the maximum of the theoretical curve, there is clearly a strong damping of the enhancement with respect to the duration T, leading to a loss of intensity at the theoretical maximum. This damping may be associated with transverse relaxation of the ¹H and ¹⁰³Rh transverse magnetisation during the polarisation transfer process.

3.2.3 ¹H–¹⁰³Rh polarisation transfer by refocused INEPT

Polarisation transfer from ¹H to ¹⁰³Rh may also be conducted by the standard refocused-INEPT pulse sequence (Burum and Ernst, 1980). In this case, the theoretical enhancement of the S-spin magnetisation, due to transfer from the I spins, is given for the I₃ S case, in the absence of relaxation and other imperfections, by Burum and Ernst (1980):

$$\begin{array}{}\text{(4)}& \begin{array}{rl}{\mathit{ϵ}}_{\text{RI}}({\mathit{\tau }}_{\mathrm{1}},{\mathit{\tau }}_{\mathrm{2}})=& {\displaystyle \frac{\mathrm{3}{\mathit{\gamma }}_{\mathrm{I}}}{\mathrm{4}{\mathit{\gamma }}_{\mathrm{S}}}}\sin \left(\mathit{\pi }{J}_{\text{IS}}{\mathit{\tau }}_{\mathrm{1}}\right)\\ & \times \left(\sin \left(\mathit{\pi }{J}_{\text{IS}}{\mathit{\tau }}_{\mathrm{2}}\right)+\sin \left(\mathrm{3}\mathit{\pi }{J}_{\text{IS}}{\mathit{\tau }}_{\mathrm{2}}\right)\right),\end{array}\end{array}$$

where τ₁ and τ₂ refer to the total echo durations including two inter-pulse intervals, as shown in Fig. 1 of Burum and Ernst (1980). The maximum of this function is found at ${\mathit{\tau }}_{\mathrm{1}}=(\mathrm{2}{J}_{\text{IS}}{)}^{-\mathrm{1}}$ and ${\mathit{\tau }}_{\mathrm{2}}=\text{arcsin}\left({\mathrm{3}}^{-\mathrm{1}/\mathrm{2}}\right)/\left(\mathit{\pi }{J}_{\text{IS}}\right)$, giving an enhancement of $\mathrm{|}{\mathit{ϵ}}_{\text{RI}}\mathrm{|}=\mathrm{|}\mathrm{2}{\mathit{\gamma }}_{\mathrm{I}}/\sqrt{\mathrm{3}}{\mathit{\gamma }}_{\mathrm{S}}\mathrm{|}$ (Doddrell et al., 1981, 1982; Pegg et al., 1981, 1982). Therefore, the maximum theoretical enhancement by refocused INEPT is

$$\begin{array}{}\text{(5)}& \left|{\mathit{ϵ}}_{\text{RI}}^{\text{max}}\right|=\mathrm{|}\mathrm{2}{\mathit{\gamma }}_{\mathrm{I}}/\sqrt{\mathrm{3}}{\mathit{\gamma }}_{\mathrm{S}}\mathrm{|}\simeq \mathrm{1.155}\mathrm{|}{\mathit{\gamma }}_{\mathrm{I}}/{\mathit{\gamma }}_{\mathrm{S}}\mathrm{|}\simeq \mathrm{36.48}\end{array}$$

for the case of I = ¹H and S = ¹⁰³Rh. Hence, in the absence of relaxation, refocused INEPT can give a slightly greater enhancement than DualPol in an I₃ S system. However, the maximum enhancements by both DualPol and INEPT are less than the theoretical bound on the enhancement of S-spin magnetisation by polarisation transfer from the I spins in a permutation-symmetric I₃ S spin system, which is equal to $\mathrm{|}\mathrm{3}{\mathit{\gamma }}_{\mathrm{I}}/\mathrm{2}{\mathit{\gamma }}_{\mathrm{S}}\mathrm{|}$ (Nielsen et al., 1995).

The theoretical advantage of INEPT over DualPol is not realised in practice for the case of ¹⁰³Rh(acac)₃. The maximum enhancement by refocused INEPT was realised for durations of τ₁ = 920 and τ₂ = 500 ms, which yielded a ¹⁰³Rh enhancement factor of ∼ 17 over thermal polarisation, i.e. less than the maximum DualPol enhancement, which was ∼ 23. The experimentally optimised interval τ₁ is significantly shorter than the optimum theoretical value in the absence of relaxation, which is ${\mathit{\tau }}_{\mathrm{1}}^{\text{theor}}$ = 1.28 s, assuming a ¹⁰³Rh–¹H spin–spin coupling of J_HRh = 0.39 Hz. The optimum value of τ₂, on the other hand, is very similar to the theoretical value, which is ${\mathit{\tau }}_{\mathrm{2}}^{\text{theor}}$ = 503 ms.

The ¹H-enhanced ¹⁰³Rh spectrum, produced by an optimised refocused-INEPT sequence, is shown in Fig. 3c. It shows a significantly lower enhancement than the DualPol result of Fig. 3b, despite the fact that the theoretical enhancement by refocused INEPT is higher than that of DualPol in the absence of relaxation (see Eqs. 3 and 5). The loss of amplitude relative to the theoretical values may be attributed to transverse ¹H relaxation during the polarisation transfer process. It is known that Hartmann–Hahn-style cross-polarisation sequences such as DualPol can outperform INEPT in the presence of transverse relaxation (Levitt, 1991).

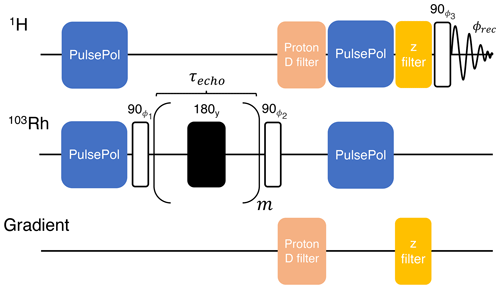
Figure 6 Sequence used for the indirect measurement of rhodium T₂ through ¹H detection. The phase cycles are given by the following: ${\mathit{\varphi }}_{\mathrm{1}}=[x,x,-x,-x]$, ${\mathit{\varphi }}_{\mathrm{2}}=[-x,x,-x,x]$, ${\mathit{\varphi }}_{\mathrm{3}}=[x,x,x,x,y,y,y,y,-x,-x,-x,-x,-y,-y,-y,-y]$ and the receiver ${\mathit{\varphi }}_{\text{rec}}=[x,-x,-x,x,y,-y,-y,y,-x,x,x,-x,-y,y,y,-y]$. The echo interval τ_echo was 45 ms. The black rectangle indicates a symmetrised BB1 composite 180° pulse (Wimperis, 1994; Cummins et al., 2003). An MLEV-64 supercycle was applied to the phases of the 180° pulses (Levitt et al., 1982a, b; Freeman et al., 1982; Gullion et al., 1990; Gullion, 1993). The ¹H “D-filter” and “z-filter” modules are described in Figs. 3 and 4 of Harbor-Collins et al. (2023), respectively.

4 ¹H-detected ¹⁰³Rh T₂

The ¹⁰³Rh T₂ relaxation time constant for ¹⁰³Rh(acac)₃ in CDCl₃ was measured via the methine ¹H signals using a variant of a previously described indirectly detected T₂ DualPol pulse sequence (Harbor-Collins et al., 2023), which is shown in Fig. 6. The pulse sequence starts with a DualPol sequence of duration T = 1.54 s to transfer thermal-equilibrium longitudinal ¹H magnetisation to ¹⁰³Rh. The ¹⁰³Rh longitudinal magnetisation is converted into ¹⁰³Rh transverse magnetisation by a 90° pulse on the ¹⁰³Rh channel. The ¹⁰³Rh transverse magnetisation evolves under a Carr–Purcell–Meiboom–Gill (CPMG) train of m spin echoes, each with an echo duration τ_echo = 45 ms. The Carr–Purcell sequence suppresses the confounding effects of translational diffusion and mixing with antiphase spin operators (Peng et al., 1991). The ¹⁰³Rh transverse magnetisation is converted to ¹⁰³Rh longitudinal magnetisation by a second 90° ¹⁰³Rh pulse. A ¹H “D-filter” module is applied to destroy any residual ¹H magnetisation, before another DualPol sequence of duration T = 1.54 s transfers the ¹⁰³Rh longitudinal magnetisation to ¹H longitudinal magnetisation. A ¹H “z-filter” module is applied to destroy any other ¹H magnetisation components, followed by a 90° ¹H pulse which excites ¹H transverse magnetisation whose precession induces a ¹H NMR signal which is detected in the following interval. The ¹H D-filter and z-filter modules are described in Figs. 3 and 4 of Harbor-Collins et al. (2023), respectively.

Repetition of the experiment with increasing values of m leads to the ¹⁰³Rh T₂ decay curve shown in Fig. 7. This fits well to a single exponential decay with the time constant T₂(¹⁰³Rh) = 18.36 ± 0.92 s.

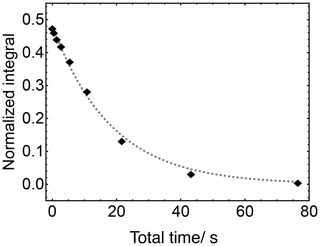
Figure 7 Decay curve for the ¹⁰³Rh transverse magnetisation of ¹⁰³Rh(acac)₃ in solution at a field of 9.4 T, measured by the indirectly detected multiple spin-echo scheme in Fig. 6. The experimental duration was 15 min.

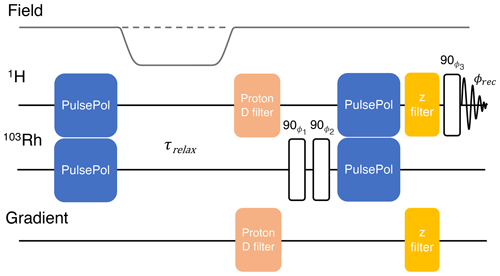
Figure 8 Sequence used for the indirect measurement of the rhodium T₁ through the ¹H NMR signal. Phase cycles are given by the following: ${\mathit{\varphi }}_{\mathrm{1}}=[x,x,-x,-x]$, ${\mathit{\varphi }}_{\mathrm{2}}=[-x,x,-x,x]$, ${\mathit{\varphi }}_{\mathrm{3}}=[x,x,x,x,y,y,y,y,-x,-x,-x,-x,-y,-y,-y,-y]$ and the receiver ${\mathit{\varphi }}_{rec}=[x,-x,-x,x,y,-y,-y,y,-x,x,x,-x,-y,y,y,-y]$. The optional shuttling of the sample to low field, and back again, during the interval τ_relax is indicated. The ¹H “D-filter” and “z-filter” modules are described in Figs. 3 and 4 of Harbor-Collins et al. (2023), respectively.

5 ¹H-detected ¹⁰³Rh T₁

The ¹⁰³Rh T₁ relaxation time constant for ¹⁰³Rh(acac)₃ in CDCl₃ was measured indirectly using the methine ¹H signals, by means of the pulse sequence shown in Fig. 8 (Harbor-Collins et al., 2023). The pulse sequence starts with a DualPol sequence of duration T = 1.54 s to transfer thermal-equilibrium longitudinal ¹H magnetisation to ¹⁰³Rh. For variable-field experiments, the sample is shuttled out of the high-field magnet into a low-field region. The nuclear magnetisation is allowed to relax for an interval τ_relax. If necessary, the sample is shuttled back into high field, and residual ¹H magnetisation is destroyed by a ¹H D-filter module. A pair of phase-cycled 90° ¹⁰³Rh pulses are applied to select for ¹⁰³Rh z-magnetisation before a second DualPol sequence of duration T = 1.54 s transfers the partially relaxed longitudinal ¹⁰³Rh magnetisation to ¹H magnetisation. A ¹H z-filter module is applied to destroy any other ¹H magnetisation components, followed by a 90° ¹H pulse which excites ¹H transverse magnetisation whose precession induces a ¹H NMR signal that is detected in the following interval. The ¹H D-filter and z-filter modules are described in Figs. 3 and 4 of Harbor-Collins et al. (2023), respectively. During τ_relax, ¹H-enhanced ¹⁰³Rh magnetisation decays toward thermal ¹⁰³Rh magnetisation, which is very small; hence, at large values of τ_relax, resulting ¹⁰³Rh-derived ¹H signals are very weak and close to zero, even for measurements performed at higher magnetic field strengths.

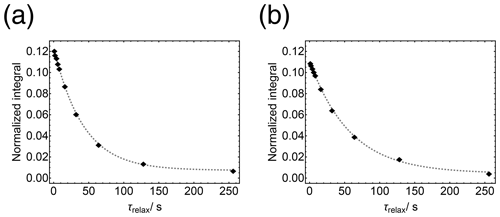
Figure 9 Trajectories of longitudinal ¹⁰³Rh magnetisation for ¹⁰³Rh(acac)₃ in solution at a temperature of 295 K, measured indirectly through the methine ¹H signals, using the pulse sequence in Fig. 8. (a) Filled symbols: ¹H signal amplitudes at a magnetic field of 9.4 T. The data were acquired in ∼ 2 h. The integrals are normalised against the ¹H spectrum obtained by a single ¹H 90° pulse applied to a system in thermal equilibrium at 9.4 T and at 295 K. Dotted line: fitted exponential decay with time constant T₁(¹⁰³Rh) = 41.8 ± 0.9 s. Panel (b) is the same as panel (a) but the sample is shuttled to a field of 10 mT during the relaxation delay τ_relax. Dotted line: fitted exponential decay with time constant T₁(¹⁰³Rh) = 57.8 ± 1.7 s.

The trajectory of indirectly detected ¹⁰³Rh z-magnetisation in a field of 9.4 T and at a temperature of 295 K is shown in Fig. 9a. The trajectory fits well to a single exponential decay with time constant T₁(¹⁰³Rh) = 41.8 ± 0.9 s. A trajectory in the low magnetic field of 10 mT and at a temperature of 295 K is shown in Fig. 9b. This was produced by shuttling the sample to a low magnetic field, and back again, during the interval τ_relax. The relaxation process is somewhat slower in a low magnetic field, with a time constant of T₁(¹⁰³Rh) = 57.8 ± 1.7 s.

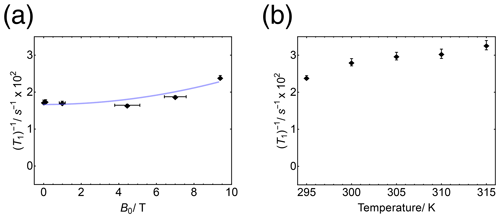
Figure 10 (a) ¹⁰³Rh relaxation rate constant $T_{\mathrm{1}}^{-\mathrm{1}}$ for ¹⁰³Rh(acac)₃ in solution, as a function of magnetic field strength at a temperature of 295 K. The blue line shows the best-fit quadratic function $T_{\mathrm{1}}^{-\mathrm{1}}\left(B\right)=T_{\mathrm{1}}^{-\mathrm{1}}\left(\mathrm{0}\right)+a{B}^{\mathrm{2}}$, where $T_{\mathrm{1}}^{-\mathrm{1}}\left(\mathrm{0}\right)$ = (167 ± 7) × 10⁻⁴ s⁻¹ and a = (7 ± 2) × 10⁻⁵ ${\mathrm{s}}^{-\mathrm{1}}{\mathrm{T}}^{-\mathrm{2}}$. (b) ¹⁰³Rh relaxation rate constant $T_{\mathrm{1}}^{-\mathrm{1}}$ for ¹⁰³Rh(acac)₃ in solution, as a function of temperature at a magnetic field strength of 9.4 T.

The observed field dependence of the ¹⁰³Rh relaxation rate constant $T_{\mathrm{1}}^{-\mathrm{1}}$ is shown in Fig. 10a. The magnetic field dependence of $T_{\mathrm{1}}^{-\mathrm{1}}$ is quite weak in this range of fields. The relaxation rate constant increases slightly with increasing magnetic field at the high-field end, suggestive of a weak relaxation contribution from the ¹⁰³Rh chemical shift anisotropy. The blue curve in Fig. 10a shows the best-fit quadratic function $T_{\mathrm{1}}^{-\mathrm{1}}\left(B\right)=T_{\mathrm{1}}^{-\mathrm{1}}\left(\mathrm{0}\right)+a{B}^{\mathrm{2}}$, where $T_{\mathrm{1}}^{-\mathrm{1}}\left(\mathrm{0}\right)$ = (167 ± 7) × 10⁻⁴ s⁻¹ and a = (7 ± 2) × 10⁻⁵ ${\mathrm{s}}^{-\mathrm{1}}{\mathrm{T}}^{-\mathrm{2}}$.

The observed temperature dependence of the ¹⁰³Rh relaxation rate constant $T_{\mathrm{1}}^{-\mathrm{1}}$ is shown for a field of B ≃ 9.4 T in Fig. 10b. The rhodium $T_{\mathrm{1}}^{-\mathrm{1}}$ increases monotonically with increasing temperature over the relevant temperature range. At 315 K, relaxation occurs with a time constant of T₁(¹⁰³Rh) = 30.6 ± 1.1 s.

A positive dependence of the ¹⁰³Rh $T_{\mathrm{1}}^{-\mathrm{1}}$ on temperature was reported previously for ¹⁰³Rh(acac)₃ in solution (Benn et al., 1985).

6 Discussion

The temperature dependence of the ¹⁰³Rh $T_{\mathrm{1}}^{-\mathrm{1}}$, as shown in Fig. 10b, indicates a dominant spin–rotation relaxation mechanism. For small molecules with a short rotational correlation time relative to the nuclear Larmor period, spin–rotation is the only mechanism that leads to a positive correlation of $T_{\mathrm{1}}^{-\mathrm{1}}$ with temperature (Hubbard, 1963). This is because the amplitudes of the local magnetic fields generated by the spin–rotation interaction are proportional to the root-mean-square rotational angular momentum of the participating molecules – a quantity that is linked to the mean rotational kinetic energy of the molecules, which increases linearly with temperature. For other mechanisms, the decrease in the rotational correlation time τ_c with increasing temperature leads to a decrease in the relaxation rate with increasing temperature, in the fast-motion limit.

The field dependence of the ¹⁰³Rh $T_{\mathrm{1}}^{-\mathrm{1}}$, as shown in Fig. 10a, displays a modest increase in relaxation rate with increasing magnetic field at high field, which suggests an additional contribution from the rotational modulation of the ¹⁰³Rh CSA tensor. A finite ¹⁰³Rh CSA tensor is allowed with respect to symmetry under the D₃ point group of ¹⁰³Rh(acac)₃ (Buckingham and Malm, 1971). Indeed, solid-state NMR data indicate a ¹⁰³Rh shielding anisotropy of Δσ ≃ 460 ppm, with relativistic quantum chemistry calculations in reasonable agreement (Holmes et al., 2023). The magnitude of this CSA tensor is modest by ¹⁰³Rh standards. For example, the ¹⁰³Rh nuclei in Rh paddlewheel complexes have a shielding anisotropy of |Δσ| ∼ 9900 ppm (Harbor-Collins et al., 2023).

In summary, we have demonstrated the successful transfer of polarisation between the central ¹⁰³Rh nucleus and the three methine ¹H nuclei in ¹⁰³Rh(acac)₃, through the very small four-bond ¹H–¹⁰³Rh couplings. The polarisation transfer is more efficient for DualPol than for refocused INEPT, even though the theoretical efficiency of DualPol is slightly less than that of refocused INEPT, for the relevant I₃ S spin system. We have successfully exploited ¹H–¹⁰³Rh polarisation transfer to study the longitudinal and transverse relaxation of ¹⁰³Rh for ¹⁰³Rh(acac)₃ in solution. The ¹⁰³Rh T₁ relaxation is dominated by the spin–rotation mechanism, with a significant additional contribution from the ¹⁰³Rh CSA at a high magnetic field.