The decay of the refocused Hahn echo in double electron–electron resonance (DEER) experiments

Double electron–electron resonance (DEER) is a pulse electron paramagnetic resonance (EPR) technique that measures distances between paramagnetic centres. It utilizes a four-pulse sequence based on the refocused Hahn spin echo. The echo decays with increasing pulse sequence length 2(τ₁+τ₂), where τ₁ and τ₂ are the two time delays. In DEER, the value of τ₂ is determined by the longest inter-spin distance that needs to be resolved, and τ₁ is adjusted to maximize the echo amplitude and, thus, sensitivity. We show experimentally that, for typical spin centres (nitroxyl, trityl, and Gd(III)) diluted in frozen protonated solvents, the largest refocused echo amplitude for a given τ₂ is obtained neither at very short τ₁ (which minimizes the pulse sequence length) nor at τ₁=τ₂ (which maximizes dynamic decoupling for a given total sequence length) but rather at τ₁ values smaller than τ₂. Large-scale spin dynamics simulations based on the coupled cluster expansion (CCE), including the electron spin and several hundred neighbouring protons, reproduce the experimentally observed behaviour almost quantitatively. They show that electron spin dephasing is driven by solvent protons via the flip-flop coupling among themselves and their hyperfine couplings to the electron spin.

1 Introduction

DEER (double electron–electron resonance) spectroscopy is a highly effective method for nanometer-scale distance measurements in bio-macromolecules such as proteins, nucleic acids, and their complexes (Jeschke, 2013; Jeschke and Polyhach, 2007). This method measures the magnetic dipolar coupling between two spin labels attached at well-defined, specific locations in the bio-macromolecules. As the dipolar coupling strength is inversely proportional to the cube of the inter-spin distance, the distance distribution between the two spin labels can be determined from the DEER signal.

The original implementation of the DEER experiment, as introduced by Milov et al. (1981, 1984), is referred to as three-pulse DEER (Fig. 1a). It employs the standard two-pulse (Hahn) echo at one frequency, ν₁, and an additional pump pulse at another frequency, ν₂, applied at time t after the first pulse. The echo amplitude is measured as a function of the pump pulse position t. This sequence suffers from instrumental artefacts near t=0 due to pulse overlap between the first pulse and the pump pulse. To eliminate these artefacts, the dead-time-free four-pulse DEER experiment was introduced by Spiess and co-workers (Fig. 1b; Martin et al., 1998; Pannier et al., 2000). This method utilizes an additional refocusing π pulse to generate the refocused echo instead of the Hahn echo, allowing the undistorted measurement of the echo around t=0. Again, the echo amplitude is measured as a function of pump pulse position, t. Currently, the four-pulse DEER sequence is the most widely used DEER experiment.

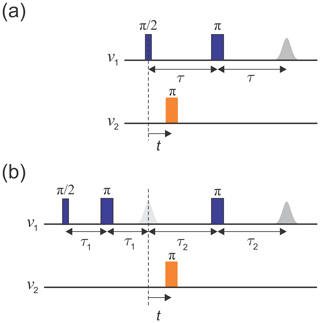
Figure 1 Schematics of (a) the three-pulse DEER sequence and (b) the dead-time-free four-pulse DEER sequence. Observer pulses are in blue and pump pulses in orange. The dashed line indicates t=0.

The echo decays as the pulse sequence length increases, and the timescale of this decay is characterized by T_M, called the phase memory time or decoherence time. Long T_M values translate into high sensitivity. The longest possible pulse sequence length that still gives a sufficient echo amplitude determines the longest distance that can be resolved. Therefore, a long T_M is required to access long distances.

The DEER signal-to-noise ratio (SNR) depends on several factors that, for the three-pulse DEER, are approximately described by Jeschke and Polyhach (2007) and Zecevic et al. (1998) as follows:

$$\begin{array}{}\text{(1)}& \text{SNR}\propto V_{\mathrm{0}}\mathit{\lambda }{\displaystyle \frac{{\mathrm{e}}^{-{\left(\mathrm{2}\mathit{\tau }/T_{\mathrm{M}}\right)}^x}}{\sqrt{T_{\mathrm{1}}}}},\end{array}$$

where V₀ is the echo intensity at τ=0 (which is proportional to the number of spins in the sample), λ is the modulation depth and represents the fraction of spins inverted by the pump pulse, x is a stretching exponent, and T₁ is the spin–lattice relaxation time, which determines the rate of the data accumulation. Equation (1) is also applied in an approximate fashion for four-pulse DEER by replacing τ with τ₁+τ₂ and setting x=1 (Jeschke and Polyhach, 2007).

According to Eq. (1), for a fixed evolution time the sensitivity decreases exponentially with decreasing T_M. Therefore, one strives to prolong T_M as much as possible. It is possible to optimize the sample to suppress some of the mechanisms that contribute to dephasing. For example, the spin concentration can be lowered to minimize dephasing contributions due to electron–electron dipolar interactions, such as spectral and instantaneous diffusion (Eaton and Eaton, 2000, 2016; Raitsimring et al., 1974). However, this concentration reduction leads to a loss in absolute signal intensity and may significantly prolong the experiment runtime, and therefore, there is an optimal concentration for the best SNR (Jeschke and Polyhach, 2007). Another mechanism that strongly contributes to dephasing is nuclear spin diffusion, which is driven by magnetic nuclei that are coupled to the electron spin and among themselves (Brown, 1979; Canarie et al., 2020; Huber et al., 2001; Lenz et al., 2017; Milov et al., 1972; Mims, 1972; Salikhov and Tsvetkov, 1979; Zecevic et al., 1998). The dephasing by this mechanism is enhanced in particular by nuclei with a large gyromagnetic ratio, such as protons. Therefore, a reduction in the proton concentration leads to a longer T_M. This can be partially achieved by using deuterated solvents, which is a common practice nowadays (Jeschke and Polyhach, 2007), and – more completely but with more effort – by also deuterating the protein (El Mkami et al., 2014; Schmidt et al., 2016). Additionally, the contribution of nuclear-spin-driven dephasing can be reduced by the application of dynamic-decoupling schemes such as the Carr–Purcell (CP) sequence (Carr and Purcell, 1954), thus prolonging T_M (Harbridge et al., 2003). This concept was behind the design of the five-pulse (Borbat et al., 2013) and seven-pulse (Spindler et al., 2015) DEER sequences, which have been shown to allow significantly longer evolution times and access to longer distances. These experiments, however, are not straightforward to run because of the contribution of unwanted transfer pathways that generate additional signal contributions to the DEER trace (Breitgoff et al., 2017). The effect of dynamic decoupling on the dephasing of paramagnetic centres in frozen solutions and in solids has been investigated in several detailed studies (Kveder et al., 2019; Ma et al., 2014; Soetbeer et al., 2018, 2021).

A common practice for DEER is to record the two-pulse echo decay to estimate the maximum evolution time that can be applied for a particular sample. However, in the case of four-pulse DEER, a refocused two-pulse echo is observed, and therefore, it is the decay of this refocused echo which determines the SNR of the experiment and the distance accessibility. In the context of DEER, it is usually assumed that the refocused echo decays monotonically as a function of the overall pulse sequence length 2(τ₁+τ₂), which is similar to the two-pulse echo. Based on this assumption, short τ₁ values are chosen to minimize dephasing during this initial interval. In this work, we show that this approach is not generally optimal, particularly in protonated solvents.

We explored the decay dependence of the refocused echo on τ₁ and τ₂, which is necessary for the optimization of the τ₁ values for achieving the best four-pulse DEER SNR. For this, we used the following three types of spin probes commonly used in DEER experiments on proteins: a common nitroxide radical, a trityl radical, and a Gd(III) complex (see Fig. 2), all in dilute frozen aqueous solutions. We show that, under conditions where the contribution of solvent nuclei to dephasing is significant (low concentrations and low temperatures), the refocused echo sequence acts as a CP-like dynamic-decoupling sequence with two π pulses (Borbat et al., 2013). In this case, under the condition of a fixed and relatively long τ₂, necessary for DEER measurements, we find that the refocused echo intensity reaches its maximum at relatively long τ₁ values that are shorter than τ₂. This is in contrast to the common use of a short τ₁, chosen based on Eq. (1) to minimize the overall pulse sequence length. We present numerical spin dynamics simulations that reproduce the experimental results almost quantitatively and provide insight into the nature of dynamic decoupling in the refocused Hahn echo.

Figure 2 Chemical structures of the paramagnetic centres studied in this work, namely (a) 3-maleimido-proxyl, (b) trityl OXO63, and (c) Gd-C2.

2 Methods

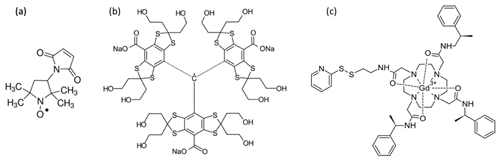
The 3-maleimido-proxyl and the trityl OXO63 were purchased from Sigma-Aldrich and from Oxford Instruments, respectively, and were used as provided. The powders were dissolved in either H₂O or D₂O to yield a 50 mM stock solution. They were further diluted using (a) a mixture of 80 % water and 20 % glycerol ($v/v$), (b) a mixture of 80 % D₂O and 20 % glycerol-d₈, or (c) mixtures thereof to create 25 %, 50 %, or 75 % deuterated solvents. The final radical concentration was 100 µM in all cases.

GdCl₃ was purchased from Sigma-Aldrich and used at a final concentration of 100 µM. The protein MdfA, labelled with Gd-C2 at positions 44 and 307 and solubilized in detergent, was prepared earlier according to a published protocol (Yardeni et al., 2019) and used without further modification. The final concentration of MdfA was about 25 µM.

Spectroscopic measurements. All measurements were carried out on a W-band (94.9 GHz), home-built spectrometer (Goldfarb et al., 2008; Mentink-Vigier et al., 2013). The two-pulse echo decays were recorded using the sequence shown in Fig. 1a, without the pump pulse, utilizing a two-step phase cycle. The two-dimensional refocused echo experiments were recorded using the sequence given in Fig. 1b, without the pump pulse, and the echo intensity was measured as a function of both τ₁ and τ₂. An eight-step-phase cycle of (x)(x)(x) was used ($+/-$x on all three pulses). Experimental parameters are listed in Table 1. In each case, the magnetic field was set to a value where the maximum of the EPR spectrum was resonant with the microwave frequency, unless stated otherwise. To produce the final signal, the echo was integrated over its full width at half maximum.

Table 1 Experimental parameters used in this work. Identical parameters were used for the two-pulse and refocused echo measurements.

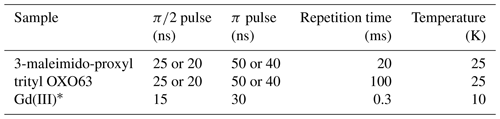
^* For both GdCl₃ and Gd-C2 labelled MdfA.

Simulations. To simulate the refocused echo decay, we follow our previously published approach (Canarie et al., 2020). A 3-maleimido-proxyl radical was geometry optimized, using density functional theory (DFT, B3LYP, and def2-SVP), and then solvated in a periodic box containing H₂O $/$ glyercol (3038 waters and 188 glycerols), using molecular dynamics. The spin system used in the spin dynamics simulation includes the unpaired electron on the radical, all protons on the radical, and all protons from H₂O and glycerol molecules within 12 Å of the electron spin (512 protons total). The spin Hamiltonian includes full nucleus–nucleus coupling tensors and the secular and pseudo-secular parts of all ${\widehat{S}}_z$–hyperfine coupling tensors (${\widehat{S}}_z{\widehat{I}}_z$, ${\widehat{S}}_z{\widehat{I}}_x$, and ${\widehat{S}}_z{\widehat{I}}_y$) calculated from the electron and nucleus positions. The echo decay was simulated by explicit fully coherent time evolution of the spin system state, using density matrix propagation in Hilbert space, without any explicit relaxation terms. The calculations were performed using a truncated ensemble cluster correlation expansion (CCE; Yang and Liu, 2008, 2009), which is a refinement of the earlier cluster expansion (Witzel and Das Sarma, 2006). Echo signals from all possible subsystems involving the electron spin and a small cluster of nuclei are calculated separately, and the resulting signals are combined to give the total signal. To limit the number of clusters, we used a maximum nuclear cluster size of 2, 3, or 4 (2-CCE, 3-CCE, 4-CCE) and neglected all clusters with intra-cluster nucleus–nucleus couplings smaller than 1.58 kHz. This gave a converged signal and included 4365 two-proton clusters and 52 937 three-proton clusters. Orientational averaging was performed over a Lebedev grid with 14 points, taking the orientation-dependent excitation efficiency of the pulses into account. The simulations used an applied magnetic field of 3.38 T.

3 Results and discussion

To investigate the refocused echo decay, we acquired its amplitude as a function of both τ₁ and τ₂ for frozen solutions of a 3-maleimido-proxyl radical, the trityl OXO063, and Gd(III) (see Fig. 2). We measured all samples in both H₂O $/$ glycerol (80:20, $v/v$) and D₂O $/$ glycerol-d₈ (80:20, $v/v$) to examine the effect of nuclear spin diffusion. This is known to be a dominant mechanism of dephasing for organic radicals in dilute frozen solutions at cryogenic temperatures (Canarie et al., 2020; Eaton and Eaton, 2000; Zecevic et al., 1998) and has been shown to be partially suppressed using dynamic decoupling (Harbridge et al., 2003; Soetbeer et al., 2018, 2021).

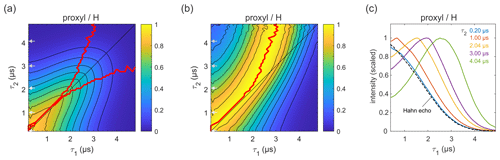
Figure 3 Refocused echo decay for 100 µM 3-maleimido-proxyl in H₂O $/$ glycerol (80:20 $v/v$) at 25 K. Panel (a) shows the echo amplitude as a function of τ₁ and τ₂, and panel (b) shows the same data after normalization of each slice along τ₁. The red lines (a, b) indicate the location of the maxima along τ₁ for fixed τ₂ (upper line) and vice versa (lower line; only in a). Panel (c) shows slices along τ₁ for several τ₂ values (indicated by grey arrows in a, b), together with a Hahn echo decay.

3.1 Organic radicals

Figure 3a presents the measured refocused echo decay data of 3-maleimido-proxyl in H₂O $/$ glycerol. It shows an overall monotonic decay as the values of τ₁ and τ₂ increase, and decay is symmetric with respect to exchange of τ₁ and τ₂. The data show that the echo decay is not a function of only the total pulse length, 2(τ₁+τ₂), which is in contrast to the two-pulse echo decay. The decay is fastest along τ₁≈0 and τ₂≈0 and slowest along the diagonal τ₁=τ₂. The latter is a manifestation of CP dynamic decoupling. While these general features of the decay are at least qualitatively as expected, the decay shape has a more subtle feature that has important practical implications for DEER measurements. In DEER, the choice of τ₂ is determined by the desired distance range, and an optimal choice of τ₁ maximizes the echo and, thereby, SNR. Optimizing τ₁ for a fixed τ₂ corresponds to finding the maximum along a particular horizontal slice of the data in Fig. 3a. This is more obvious in Fig. 3b, which shows the data from Fig. 3a after individual normalization of each horizontal τ₁-dependent trace with fixed τ₂. The superimposed red curve represent the loci of the maxima along these slices, corresponding to optimal τ₁ values for DEER. A similar plot and curve can be generated for vertical slices (fixed τ₁; variable τ₂), due to the symmetry of the decay across the τ₁=τ₂ diagonal. The curves are also superimposed in Fig. 3a. The crucial feature of theses curves is their deviation from the diagonal (τ₁=τ₂) for increasing values of τ₁ and τ₂.

Figure 3c shows individual slices at several τ₂ values (indicated in Fig. 3a and b by grey arrows), together with a two-pulse echo decay (dashed line) as reference. This shows that, in protonated solvents, the refocused echo decay along τ₁ (and, by symmetry, along τ₂) cannot generally be described by a stretched exponential decay. While there is little difference between the refocused echo decay and the two-pulse echo decay for small τ₂ values, for larger τ₂ values the refocused echo first grows significantly from its short-τ₁ amplitude, forms a broad maximum, and only then starts decaying. As a general trend, the longer the τ₂, the more pronounced the effect. This is relevant for DEER experiments, which are usually run with long τ₂ values to provide long dipolar evolution times. Interestingly, the maximum intensity does not appear at τ₁=τ₂, where it would match the CP condition of maximal dynamic decoupling. Nor does it occur at τ₁≈0, corresponding to the minimal total pulse sequence length. Rather, it is slightly detuned from the CP diagonal and appears at τ₁<τ₂. This deviation from the diagonal increases with increasing τ₂ values. In practice, for a DEER experiment in protonated solvents, this shows that setting τ₁ to a short value (to minimize total pulse sequence length), or equal to τ₂ (to maximize dynamic decoupling), leads to a significant and unnecessary loss of sensitivity. We observed this identical behaviour also at a magnetic field set to the region of g_zz, as shown (and in Fig. S1a–b in the Supplement). We also checked that the contributions of instantaneous diffusion under these conditions were negligible by comparing the Hahn echo decay obtained with different pulse lengths (see Fig. S1c).

Figure 4 Refocused echo decay for 100 µM 3-maleimido-proxyl in D₂O $/$ glycerol-d₈ (80:20 $v/v$) at 25 K. Panel (a) shows the echo amplitude as a function of τ₁ and τ₂, and panel (b) shows the same data after normalization of each slice along τ₁. Panel (c) shows slices along τ₁ for several τ₂ values (indicated by grey arrows in a, b), together with a Hahn echo decay.

Figure 4a shows the refocused echo decay for 3-maleimido-proxyl in the corresponding deuterated solvent. Here, both the Hahn echo and the refocused echo decays are significantly extended as compared to the protonated solvent, as expected, due to the absence of protons in the sample. The effect observed in protonated solvent is practically absent here. The echo amplitude depends only on the total pulse sequence length, 2(τ₁+τ₂). The decays along τ₁ for fixed τ₂ are essentially independent of τ₂ and resemble very much the two-pulse echo decay (see Fig. 4b–c). It is apparent that nuclear spin diffusion is suppressed here, and dynamic decoupling is ineffective. The decay is dominated by other dephasing mechanisms such as instantaneous diffusion (Raitsimring et al., 1974) and therefore, a short τ₁ gives optimal sensitivity. It has recently been reported that, for a deuterated nitroxide attached to a model compound dissolved in 50:50 ($v/v$) D₂O $/$ glycerol-d₈ or in deuterated o-terphenyl (OTP), the dynamic decoupling was efficient (Soetbeer et al., 2018, 2021). In this case, the protons on the large model compound generate a significant proton concentration in the vicinity of the nitroxide, which contributes to dephasing. We also note that the Hahn echo decays we observed do not reveal the significant fast echo decay component detected in the protonated and deuterated nitroxides in D₂O $/$ glycerol-d₈ at low temperatures (10–50 K) that were reported recently (Soetbeer et al., 2021).

Figure 5 Refocused echo decay for 100 µM 3-maleimido-proxyl in H₂O $/$ glycerol solvents (80:20 $v/v$) with varying degrees of solvent protonation at 25 K, i.e. (a) 25 %, (b) 50 %, and (c) 75 %. The red lines indicate the location of the maxima along τ₁ for fixed τ₂ (upper line) and vice versa (lower line). Plots for 100 % and 0 % solvent protonation are shown in Figs. 3a and 4a, respectively.

In order to evaluate how the degree of protonation affects the refocused echo decay shape, we measured samples in a partially deuterated solvent with 25 %, 50 %, and 75 % protonation. The results are shown in Fig. 5. These measurements reveal that, already at 25 % protonation, there is a strong impact on the decay timescale and shape, as can be seen by comparing Fig. 5a to Fig. 4a. At 50 % protonation, the effect is close to complete (Fig. 5b and c compared to Fig. 3a). The T_M values determined from two-pulse echo decays, given in Table 2, show a similar trend. They decrease with increasing proton concentration. The impact is strongest when going from 0 % protonation to 25 % and 50 % protonation. The differences between 50 %, 75 %, and 100 % protonation are smaller.

Table 2 The phase memory time, T_M, and the stretching exponent, x, obtained from fitting stretched exponential decays to the two-pulse echo decays (25 K) of the 3-maleimido-proxyl samples with different degrees of solvent protonation.

Figure 6 Refocused echo decays for 100 µM trityl OXO63 in (a) H₂O $/$ glycerol (80:20 $v/v$) and (b) D₂O $/$ glycerol-d₈ at 25 K; in panel (a), the red lines show the position of the echo maximum along τ₁ for each constant τ₂ (upper line) and vice versa (lower line). Panel (c) shows slices of the data in panel (b) along τ₁ for several τ₂ values (indicated by grey arrows in b), together with a Hahn echo decay.

Figure 6 shows refocused echo decay data of the trityl OXO63, which is also used as a spin label for DEER on proteins and nucleic acids (Yang et al., 2012; Reginsson et al., 2012; Giannoulis et al., 2019). The data reveal the same behaviour as that observed for 3-maleimido-proxyl. Again, in a fully protonated solvent (Fig. 6a), the decay is fastest along τ₂≈0 and τ₁≈0, and it is slowest along the diagonal τ₁=τ₂. The timescale is very similar to that of the 3-maleimido-proxyl decay. The asymmetry at short τ₁ and τ₂ is an experimental imperfection. Theoretically, in the high-temperature limit (which is applicable here), with ideal pulses (neglecting intra-pulse evolution), and for T₁≫T_M (applicable here), one can show that the echo intensity is symmetric with respect to τ₁ and τ₂. As for 3-maleimido-proxyl, the location of the slice-wise maximum echo intensities (indicated by red lines) again deviates from the CP diagonal as τ₂ (or τ₁) increases. In contrast, in a fully deuterated solvent (Fig. 6b), no maxima in the echo intensities are observed, although there is a clear deviation from a pure (τ₁+τ₂)-dependent decay as compared to 3-maleimido-proxyl in a deuterated solvent (Fig. 4). Figure 6c clearly shows that, as τ₂ increases, the echo intensity as a function of τ₁ persists longer. This indicates that nuclear spin diffusion, induced by the trityl OXO63 protons themselves, as reported recently (Soetbeer et al., 2021), is still a contributing mechanism, though not dominant enough to result in a non-monotonic behaviour as a function of τ₁.

3.2 Gd(III)

Next, we carried out similar measurements on high-spin Gd(III) ($S=\mathrm{7}/\mathrm{2}$), as Gd(III)–Gd(III) DEER on proteins is becoming more common (Feintuch et al., 2015). The results for GdCl₃ in protonated and deuterated solvents are shown in Fig. 7. In both solvents, the decay is essentially symmetric with respect to τ₁ and τ₂. In a protonated solvent (Fig. 7a), the shape of the 2D decay is generally similar to the ones observed for 3-maleimido-proxyl and trityl OXO63, except for short τ₁ and τ₂ with slice-wise echo maxima detuned from the CP condition (red lines). For small τ₂ values below about 1.5 µs, the optimal τ₁ is as short as possible, indicating that a second dephasing mechanism, in addition to nuclear spin diffusion, such as the transient zero field splitting mechanism, is contributing (Raitsimring et al., 2014). This is quite different from the organic radicals. In a deuterated solvent (Fig. 7b), the decay more closely resembles those observed for the organic radicals (Figs. 4a and 6b). As evident from Fig. 7c, proton nuclear spin diffusion, arising from protons on the Gd(III) ligand, plays a role in dephasing that is lower than in trityl OXO63 (Fig. 6c) but higher than in 3-maleimido-proxyl (Fig. 4c).

In all cases studied, a particularly interesting observation is the deviation of the maximum echo from the diagonal (the red lines in Figs. 3a, 5a, 6a, and 7a). For 3-maleimido-proxyl, the point at which the maxima starts to deviate from the diagonal is around τ₁=1.5 µs for 100 % protonation and increases to about 2.7 µs for 25 % protonation. The deviation point for trityl OXO63 is similar, but for Gd(III) the behaviour is different, and the maximum echo is never observed along the diagonal, even for short τ₁ and τ₂. This general non-monotonic behaviour contrasts with the usual assumption of a monotonic decay and is rationalized as a balance between two competing effects that occur as τ₁ is increased from 0 to τ₂, leading to increased dephasing due to extending the pulse sequence length, on the one hand, and, on the other hand, the gain in amplitude due to the increasing degree of dynamic decoupling as τ₁ approaches τ₂.

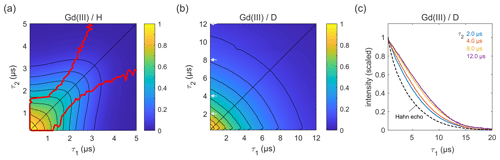
Figure 7 Refocused echo decays for 100 µM GdCl₃ in (a) H₂O $/$ glycerol (80:20 $v/v$) and (b) D₂O $/$ glycerol-d₈ at 10 K; in panel (a), the red lines show the position of the echo maximum along τ₁ for each constant τ₂ (upper line) and vice versa (lower line). Grey arrows in (b) indicate the τ₂ values of slices shown in (c), together with a Hahn echo decay.

Most applications of DEER are carried out on samples with a deuterated solvent, typically a mixture of D₂O $/$ buffer and glycerol-d₈. However, complete deuteration is rarely achieved, as the buffer, substrates, detergent, lipid membrane, and the protein or nucleic acid of interest contain non-exchangeable protons that can be in close proximity to the spin label. There are also cases in which proteins precipitate in D₂O (Verheul et al., 1998; Reslan and Kayser, 2018). As it is now clear that the echo decay is strongly influenced by the presence of even small amounts of protons in the sample, it is still advisable to optimize the value of τ₁, even if the sample is only partially protonated. An example for a sample with incomplete deuteration is the membrane protein MdfA V44C/V307C, doubly labelled with the Gd-C2 tag, where labelling positions are on the exposed periplasmic face of the protein (Yardeni et al., 2019). MdfA is protonated and, being a membrane protein, is solubilized in detergent (n-dodecyl-β-D-maltopyranoside – DDM) micelles, which have a long alkyl chain with non-exchangeable protons. Consequently, even at 100 % solvent deuteration, a significant fraction of protons is present in the sample, in close proximity to the labels. These protons are expected to affect dephasing, as noted earlier for spin labels in micelles and lipid bilayers (Dastvan et al., 2010). Figure 8 shows the measured refocused echo decay as a function of τ₁ for selected fixed τ₂ times. The non-monotonic behaviour at the largest τ₂ is qualitatively similar to that observed for the organic radicals and GdCl₃. While the maximum is not as pronounced, this behaviour still has practical implications. The dipolar evolution time of DEER is typically in the range of 3–4 µs (yellow and purple traces), so τ₁ can safely be extended to almost 3 µs without a loss of sensitivity. This was exploited, for example, to remove instrumental artefacts from the DEER trace when shaped pulses are used (Bahrenberg et al., 2019).

Figure 8 Refocused echo decay experiments (constant τ₂; variable τ₁) on 25 µM MdfA V44C/V307C, doubly labelled with Gd-C2, at 10 K. Selected values for τ₂ in nanoseconds are colour coded. Data taken from the Supplement of Yardeni et al. (2019).

3.3 Simulations

To better understand the physical origin of the observed refocused echo decay, we performed a numerical quantum spin dynamics simulation for a 3-maleimido-proxyl radical solvated in H₂O $/$ glycerol as a representative of the behaviour observed experimentally. The molecular and solvation geometries were determined by DFT and molecular dynamics, respectively. The fully coherent spin dynamics simulation included the unpaired electron on the radical and all 512 protons within 12 Å of the unpaired electron. All ${\widehat{S}}_z$–hyperfine and nucleus–nucleus coupling terms were included in the spin Hamiltonian. To handle the large Hilbert space with 2⁵¹³ spin states, a truncated ensemble correlated cluster expansion (CCE) approach was utilized (see Sect. 2). This methodology has recently been shown to accurately reproduce the two-pulse echo decays of organic radicals in frozen, dilute protonated solutions (Canarie et al., 2020).

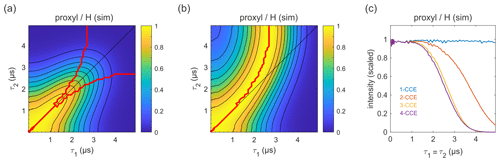
Figure 9 Simulation of the refocused echo decay for 3-maleimido-proxyl in H₂O $/$ glycerol (80:20 $v/v$). Panel (a) shows the simulated refocused echo amplitude as a function of τ₁ and τ₂, using 3-CCE. The τ₁=τ₂ line is shown in black. The upper red curve in panel (a) indicates the ridge of panel (b), which normalizes each slice along τ₁ (with constant τ₂) to unit maximal amplitude. The lower red curve in panel (a) is the analogous ridge for normalization along τ₂. Panel (c) shows the simulated refocused echo decay for τ₁=τ₂ as a function of cluster truncation level (1-CCE through 4-CCE).

The simulated refocused echo decay is shown in Fig. 9a and b. Remarkably, it almost quantitatively matches the experimental result (Fig. 3a and b) both in shape and timescale, in particular with respect to the deviation of the maxima from the diagonal for long τ₁ and τ₂. A direct comparison with the experiment is shown in Fig. S2, where the largest deviation of the theory from experiment occurs for very short τ₂. The simulations show that the only terms in the spin Hamiltonian affecting the decay are the secular parts of the hyperfine couplings and the flip-flop terms of the nucleus–nucleus coupling (data not shown). In contrast, if the nucleus–nucleus couplings are neglected, the effect disappears. This is shown in Fig. 9c; if only one-nucleus clusters are included, namely, all nucleus–nucleus couplings are neglected, then no decay is seen. The shallow modulations are due to pseudo-secular components of the hyperfine couplings. Including two-nucleus clusters in the simulation yields an echo decay that has the correct shape and a timescale of the correct order of magnitude. Adding three-nucleus clusters improves the timescale slightly, and including larger clusters does not lead to further improvements. Water and H₂O $/$ glycerol have very similar proton concentrations, so the decays are expected to be similar. Indeed, simulations comparing water and H₂O $/$ glycerol mixtures show that the two matrices give very similar results (see Fig. S3).

The conceptual essence of the mechanism can be pictured with one electron and a pair of nuclei, all coupled among each other. The associated three-spin system has eight eigenstates, with four nuclear eigenstates in each of the two electron spin manifolds (α and β). Due to presence of the flip-flop term of the nucleus–nucleus coupling, the nuclear eigenstates in the α manifold are different from those in the β manifold (assuming the two hyperfine couplings are not identical), and formally forbidden EPR transitions with Δm_I≠0 have non-zero transition amplitudes. Excitation of the system from one of its eigenstates in one manifold into the other manifold therefore generates nuclear coherence. This results in a periodic modulation of the electron spin echo amplitude as a function of inter-pulse delays, in a fashion analogous to electron spin echo envelope modulation (ESEEM; Van Doorslaer, 2017; Schweiger and Jeschke, 2001). Every cluster of nuclei contributes such a periodic modulation to the overall echo, with varying amplitudes and frequencies, depending on the structure of the cluster and its location relative to the electron spin. The solvent environment of the electron spin on the radical contains many nuclear clusters, and the echo modulations from all clusters combine to give an overall echo decay. Although the echo decays, the coherence lives on until it is destroyed by electron and nuclear T₁ relaxation processes.

Traditionally, this dephasing mechanism has been explained in terms of a stochastic nuclear spin diffusion model that involves flip-flop events between pairs of nuclei with a phenomenological flip-flop rate constant (Milov et al., 1973; Zecevic et al., 1998). However, the first-principles simulation shown earlier (Canarie et al., 2020) and here reveals that the term diffusion might not be entirely appropriate, as the quantum model that reproduces the echo decays is fully coherent and does not contain any relaxation terms or other stochastic elements. It might, therefore, be conceptually more accurate to refer to this dephasing mechanism as nuclear-spin-bath-driven electron spin decoherence, although this is clearly more tedious. It has been shown that the effect is field independent (no change between X and Q bands; Canarie et al., 2020). Also, theoretical considerations of a simple system of one electron spin and two spin-$\mathrm{1}/\mathrm{2}$ nuclei (Witzel and Das Sarma, 2006) show that the effect is independent of the field and depends only on the ratio of the nucleus–nucleus coupling to the difference in the two hyperfine couplings. We demonstrate this also for the refocused echo decay, where we compare simulations for W band versus Q band (see Fig. S4), which show that the only difference is in the ESEEM modulations, and that the nuclear-spin-bath-driven dephasing is field independent.

The dynamic decoupling effect in the refocused echo decay, i.e. the decoherence suppression along τ₁=τ₂, can be understood a little better with the simplified model Hamiltonian as follows:

$$\begin{array}{}\text{(2)}& \begin{array}{rl}\widehat{H}& ={\mathit{\mu }}_{\mathrm{B}}{g}_{\mathrm{e}}{B}_{\mathrm{0}}{\widehat{S}}_z+\textcolor{red}{}\sum _n\left(-{\mathit{\mu }}_{\mathrm{N}}{g}_n{B}_{\mathrm{0}}{\widehat{I}}_{zn}+A_n{\widehat{S}}_z{\widehat{I}}_{zn}\right)\\ & +\textcolor{red}{}\sum _{m<n}{b}_{mn}\left({\widehat{I}}_{+m}{\widehat{I}}_{-n}+{\widehat{I}}_{-m}{\widehat{I}}_{+n}-\mathrm{4}{\widehat{I}}_{zm}{\widehat{I}}_{zn}\right),\end{array}\end{array}$$

where all symbols have their usual meaning. In particular, A_n represents the secular hyperfine coupling of nucleus n. The last sum contains the secular and flip-flop components of the couplings between nuclei m and n, with coupling parameter b_nm. Note that, in this Hamiltonian, the pseudo-secular hyperfine terms are omitted; however, they are included in all CCE simulations. Calculating the refocused echo amplitude V(τ₁,τ₂), using density matrix propagation with ideal pulses, and Taylor expanding the resulting expression in terms of b_mn gives a power series where the lowest order non-vanishing term is second order in b_mn as follows:

$$\begin{array}{}\text{(3)}& \langle V_{\mathrm{2}}\rangle =-\sum _{m\ne n}{\displaystyle \frac{\mathrm{2}{b}_{mn}^{\mathrm{2}}}{{\mathit{\omega }}_{mn}^{\mathrm{2}}}}{\left[\cos \left({\mathit{\omega }}_{mn}{\mathit{\tau }}_{\mathrm{1}}\right)-\cos \left({\mathit{\omega }}_{mn}{\mathit{\tau }}_{\mathrm{2}}\right)\right]}^{\mathrm{2}},\end{array}$$

where ${\mathit{\omega }}_{mn}=(A_m-A_n)/\mathrm{2}$. This term is negative for τ₁≠τ₂ and zero for τ₁=τ₂. 〈V₂〉 also occurs as a factor to all higher-order terms in the power series. Factoring these out gives the power series for the exponential function, so that 〈V₂〉 contributes a factor of ${\mathrm{e}}^{\langle V_{\mathrm{2}}\rangle }$ to the overall echo amplitude. In general, each term in the Taylor series can be factored into terms that can be collected into exponentials, yielding the following:

$$\begin{array}{}\text{(4)}& V\left({\mathit{\tau }}_{\mathrm{1}},{\mathit{\tau }}_{\mathrm{2}}\right)={\mathrm{e}}^{\langle V_{\mathrm{2}}\rangle +\langle V_{\mathrm{3}}\rangle +\mathrm{\dots }}={\mathrm{e}}^{\langle V_{\mathrm{2}}\rangle }\cdot f\left({\mathit{\tau }}_{\mathrm{1}},{\mathit{\tau }}_{\mathrm{2}}\right),\end{array}$$

where 〈V₃〉 is the term cubic in b_mn, and we have collected all terms of orders higher than 2 in b_nm into a single term, f. This factorization is referred to as the linked-cluster expansion (Saikin et al., 2007).

Under the CP condition of τ₁=τ₂, 〈V₂〉 vanishes and, therefore, ${\mathrm{e}}^{\langle V_{\mathrm{2}}\rangle }=\mathrm{1}$. The third-order term ${\mathrm{e}}^{\langle V_{\mathrm{3}}\rangle }$ is negligible, leaving the fourth-order term as the lowest non-trivial term (Ma et al., 2014). Deviating from the τ₁=τ₂ line introduces a non-zero negative second-order term and, therefore, ${\mathrm{e}}^{\langle V_{\mathrm{2}}\rangle }<\mathrm{1}$. The significant three-spin terms are at least fourth order in b_nm and so are less significant when the second-order term contributes. This partially explains why three clusters in the simulations compress V(τ₁,τ₂) along the τ₁=τ₂ line – the CP effect breaks down at the same order that the three clusters start to contribute (Ma et al., 2014).

Maximizing echo intensity at a given τ₂ requires determining a τ₁ value that balances the loss from that which is deviating from the CP condition (quadratic in b_nm) with the loss from a long total experiment (quartic in b_nm).

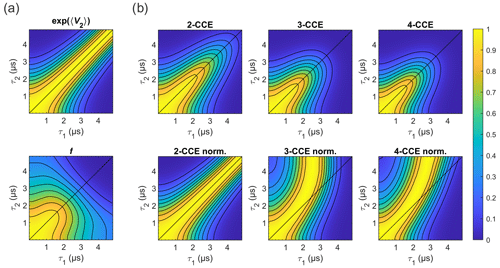
Figure 10 Simulated refocused echo decay of 3-maleimido-proxyl. (a) Factorization of the four-cluster decay into a second-order term in b_nm (top) and all higher-order terms (bottom). (b) Simulated refocused echo decays at two-, three-, and four-cluster level (2-CCE, 3-CCE, and 4-CCE; top), with the corresponding slice-wise normalized decays (bottom). For this simulation, a single orientation of the radical was used.

Figure 10 illustrates the two factors ${\mathrm{e}}^{\langle V_{\mathrm{2}}\rangle }$ and f for the 3-maleimido-proxyl case and CCE simulations up to 4-CCE in both standard and slice-wise normalized forms. It can be seen that ${\mathrm{e}}^{\langle V_{\mathrm{2}}\rangle }$ accounts for most of the CP effect (Fig. 10a; top) that f represents, predominantly, the decay along τ₁+τ₂ (Fig. 10a; bottom), and that 3-nucleus clusters contribute most to the part of f that drives the slice-wise maximum away from τ₁=τ₂ (slice-wise diagrams; Fig. 10b; bottom).

Using the calculated shapes of ${\mathrm{e}}^{\langle V_{\mathrm{2}}\rangle }$ and f in Fig. 10, we can rationalize the existence of a maximum of V(τ₁,τ₂) along τ₁ for fixed τ₂, i.e. why we observe $\partial V/\partial {\mathit{\tau }}_{\mathrm{1}}=\mathrm{0}$ for some $\mathrm{0}<{\mathit{\tau }}_{\mathrm{1}}<{\mathit{\tau }}_{\mathrm{2}}$. The derivative is as follows:

$$\begin{array}{}\text{(5)}& {\displaystyle \frac{\partial V({\mathit{\tau }}_{\mathrm{1}},{\mathit{\tau }}_{\mathrm{2}})}{\partial {\mathit{\tau }}_{\mathrm{1}}}}={\mathrm{e}}^{\langle V_{\mathrm{2}}\rangle }{\displaystyle \frac{\partial f}{\partial {\mathit{\tau }}_{\mathrm{1}}}}+V\left({\mathit{\tau }}_{\mathrm{1}},{\mathit{\tau }}_{\mathrm{2}}\right){\displaystyle \frac{\partial \langle V_{\mathrm{2}}\rangle }{\partial {\mathit{\tau }}_{\mathrm{1}}}}.\end{array}$$

At τ₁=τ₂, both derivatives on the right-hand side are negative (as seen in Fig. 10), rendering $\partial V/\partial {\mathit{\tau }}_{\mathrm{1}}$ negative – increasing τ₁ beyond τ₂ always decreases the echo, since the CP suppression is lost and the total evolution time becomes longer. In addition, for a given τ₂, and for some region τ₁<τ₂, ${\mathrm{e}}^{\langle V_{\mathrm{2}}\rangle }$ grows more rapidly with τ₁ than f decays with τ₁, as seen in Fig. 10. Therefore, $\partial V/\partial {\mathit{\tau }}_{\mathrm{1}}>\mathrm{0}$, which indicates that there is at least one τ₁ for which an increase in τ₁ increases V. This is related to the Taylor series converging fast enough. An increase in V with τ₁ indicates the gain in echo amplitude from approaching the CP condition is larger than the loss from prolonging the total evolution time. Taken together, this means that $\partial V/\partial {\mathit{\tau }}_{\mathrm{1}}$ must be zero for some τ₁<τ₂ and, therefore, V maximal. At that point, the effects of increasing ${\mathrm{e}}^{\langle V_{\mathrm{2}}\rangle }$ (signal gain due to better dynamic decoupling) and decreasing f (signal loss due to longer evolution time) are balanced.

4 Conclusions

We observe that, for low-concentration nitroxide, trityl and Gd(III) paramagnetic centres in protonated solvents, where the so-called nuclear spin diffusion decoherence mechanism dominates, the refocused two-pulse echo amplitude as a function of τ₁ for a fixed τ₂ was neither maximal for τ₁=0 (which minimizes total pulse sequence duration) nor τ₁=τ₂ (which maximizes dynamic decoupling given a fixed total pulse sequence duration) but rather for a τ₁ value between 0 and τ₂. We observed this effect in samples with 25 %–100 % solvent protonation. In fully deuterated solvents, the effect was not observed owing to fact that other dephasing mechanisms (such as instantaneous diffusion) become significant or dominant, at least at the concentrations employed in this study.

First-principle spin dynamics simulations, using a solvated nitroxide radical structure, reproduced both the timescale and the shape of the observed refocused echo decay, indicating that it is due to the large number of protons proximal to the spin label. This confirms that nuclear-spin-driven decoherence is the main mechanism of echo decay in the protonated samples under the conditions investigated (low concentration and low temperature). Although in this work we focused on the decay of the refocused echo as a function of the time intervals τ₁ and τ₂, it is interesting to compare our Hahn echo decay shapes with those reported earlier by Soetbeer et al. (2018), where the data were analysed in terms of a sum of two stretched exponentials – one with a fast decay and another with a slow decay. We do not observe the fast decay, which was particularly prominent in the protonated and deuterated nitroxides in D₂O $/$ glycerol-d₈ at low temperatures (10–50 K; Soetbeer et al., 2021). Similarly, this was not observed in our earlier report on trityl, nitroxide, and Gd(III) spin labels as being free and attached to a protein (Yang et al., 2020). The difference could be due to the field (Q band vs W band) or the different type of nitroxide used.

These findings have practical implications for DEER experiments. There, τ₂ is typically determined by structural considerations, such as the longest distances that need to be resolved. The choice of τ₁ that maximizes SNR for a given τ₂ is therefore important. Our results indicate that it is important to explore the entire range of possible τ₁ values in order to find the maximum SNR for the DEER measurement, in particular for samples that cannot be produced with 100 % deuteration of all components (solvent, protein, detergent, etc.).